Integration using polar coordinates
Integration using polar coordinates is a technique for solving integrals using polar coordinates. Sometimes an integral that is complicated in one set or coordinates, such as Cartesian coordinates become very easy or even trivial in polar coordinates.
Example
Consider the integral
This can be converted into polar coordinated by multiplying 
by itself, so that
This can be expressed as the double integral,
Now the usefulness of polar coordinates becomes apparent as in polar coordinates,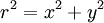 
. The bounds mean the integral is over the entire x-y plane, so 
varies from 
to 
and 
from to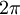 
. To convert the differentials, we must multiply by the Jacobian, to get
This can be integrated by separating the integral. The integral gives 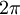and the integral gives 
. This means the initial integral, is 
.
