Difference between revisions of "Manifold"

From Conservapedia
Jump to: navigation, search
(→‎Constructing new manifolds from old: Spelling/Grammar Check & Cleanup)
 
(6 intermediate revisions by 6 users not shown)
Line 1: Line 1:
 
[[Image:Mobius.png|right|thumb|The [[Möbius strip]] is an example of a 2-manifold.]]
 
[[Image:Mobius.png|right|thumb|The [[Möbius strip]] is an example of a 2-manifold.]]
−
An ''n''-dimensional manifold (or ''n''-manifold) ''M'' is a [[topological space]] such that every point in ''M'' has a neighbourhood ''U'' that is [[homeomorphism|homeomorphic]] to ''R''<sup>''n''</sup>. The homeomorphisms <math>\phi_U : U\rightarrow \mathbf{R}^n</math> should be thought of as providing local coordinates in the neighborhood ''U''. Whenever two such coordinate neighborhoods ''U'' and ''V'' intersect, we also require that the change of coordinate maps <math>\phi_U\circ\phi_V^{-1}:\phi_V(U\cap V)\rightarrow \phi_U(U\cap V)</math> be homeomorphisms.
+
An ''n''-[[dimension]]al '''manifold''' (or ''n''-manifold) ''M'' is a [[topological space]] such that every point in ''M'' has a [[neighbourhood]] that is [[homeomorphism|homeomorphic]] to <math>\mathbb{R}^n</math>. These homeomorphisms induce a [[coordinatization]] of ''M'', and it is further required that the coordinatization is continuous.
  
−
An ''n''-dimensional differentiable manifold is an ''n''-manifold with the property that the change of coordinate maps are differentiable functions.
+
An alternate definition constructs the manifolds over the [[complex numbers]] instead of the [[real numbers]]. An ''n''-dimensional complex manifold ''N'' is a [[topological space]] such that every point in ''N'' has a neighbourhood that is homeomorphic to '''C'''<sup>''n''</sup> and whose coordinatization by these homeomorphisms is [[holomorphic]] ([[analytic]]).
−
 
+
−
An ''n''-dimensional complex manifold ''N'' is a [[topological space]] such that every point in ''N'' has a neighbourhood that is [[homeomorphism|homeomorphic]] to '''C'''<sup>''n''</sup>, and such that the change of coordinate functions are holomorphic.
+
  
 
Manifolds are [[Hausdorff space|Hausdorff]] and [[2nd-countable space|2nd-countable]].
 
Manifolds are [[Hausdorff space|Hausdorff]] and [[2nd-countable space|2nd-countable]].
  
−
Example: Consider the set of points <math>[x_0:x_1:x_2]</math>, where <math>x_i</math> are not all zero, and where we declare <math>[x_0:x_1:x_2]</math> to be equivalent to <math>[y_0:y_1:y_2]</math> if <math>x_i = \lambda y_i</math>. This space describes the set <math>\mathbf{R}P^2</math> of all one-dimensional subspaces of <math>R^3</math>. Let <math>U_i</math> be the open set with <math>x_i \neq 0</math>. Define <math>\phi_0: U_0\rightarrow R^2</math> to be the map given by <math>[x_0:x_1:x_2]\mapsto (\frac{x_1}{x_0},\frac{x_2}{x_0})</math>. Define <math>\phi_1</math> and <math>\phi_2</math> similarly. Then <math>\phi_i</math> clearly define homeomorphisms from <math>U_i</math> to <math>R^2</math>. It is simple to verify that the change of coordinate functions are also diffeomorphisms. Hence <math>\mathbf{R}P^2</math> is a differentiable manifold. In fact, <math>\mathbf{R}P^2</math> with a disk removed actually defines the mobius strip.
+
==Constructing new manifolds from old==
 +
 
 +
*Suppose that <math>f:R^n \rightarrow R^m</math> is a differentiable function. Then <math>f^{-1}(y)</math> is a smooth manifold if ''y'' is a regular value of ''f''.
 +
 
 +
[[Category:Topology]]
 
[[Category:Mathematics]]
 
[[Category:Mathematics]]
−
[[category: Topology]]
 

Latest revision as of 17:39, June 29, 2016

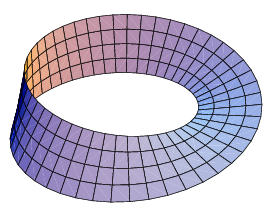
The Möbius strip is an example of a 2-manifold.

An n-dimensional manifold (or n-manifold) M is a topological space such that every point in M has a neighbourhood that is homeomorphic to . These homeomorphisms induce a coordinatization of M, and it is further required that the coordinatization is continuous.

An alternate definition constructs the manifolds over the complex numbers instead of the real numbers. An n-dimensional complex manifold N is a topological space such that every point in N has a neighbourhood that is homeomorphic to Cn and whose coordinatization by these homeomorphisms is holomorphic (analytic).

Manifolds are Hausdorff and 2nd-countable.

Constructing new manifolds from old

  • Suppose that is a differentiable function. Then is a smooth manifold if y is a regular value of f.
Retrieved from "https://conservapedia.com/index.php?title=Manifold&oldid=1243122"