SUVAT equations

The SUVAT equations are five equations that describe the motion of a body with constant linear acceleration. The five equations are:

where:

is the final velocity

is initial velocity

is the position of the body at time

is the initial position of the body

is acceleration

It is important to note that these equations can only be used if acceleration is constant, otherwise integration must be used.

Derivation

The first two SUVAT equations can be derived using integration. The others can be found by substituting one SUVAT equation into another to remove a variable.

First Equation

The acceleration of a body is the rate of change (the derivative) of its speed. Therefore, the we can integrate the acceleration to find the speed at some time as:

The boundary conditions are that at time is zero, the initial speed of the body is u and at time equal to t, the speed is v. Note that is a function that describes the acceleration of the body, while the on the right hand side of the equation is a constant. Integrating produces the first SUVAT equation:

Second Equation

The speed of a body is the rate of change of its position. The first SUVAT equation can be integrated to obtain the position of the body. Using that the position of the body is s₀ at time 0 and s at time t, then:

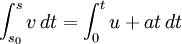
This produces the second SUVAT equation:

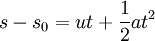
Third Equation

If 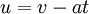is used instead of in the derivation of the second equation, then the third SUVAT equation is produced:

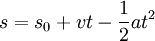
Fourth Equation

If the second and third SUVAT equations are added together, then the result is:

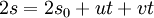
Dividing by 2 and taking out a factor of t produces the fourth SUVAT equation:

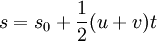
Fifth Equation

The fourth equation can be rearranged to give:

Substituting this into the first equation gives the fifth and final SUVAT equation.

Example

As the SUVAT equations are only valid for constant acceleration, their use is very limited. However, they can be applied to projectile motion, if the motion is close to the earth's surface and air resistance can be ignored. This can be done as close to the earth's surface, the acceleration due to gravity is approximately constant and takes the value -g in the y direction and 0 in the x direction. If a particle is launched with an initial speed u, at an angle θ above the horizontal and at the origin, the second SUVAT equation can be used to find the x and y positions of the particle at a later time:

These two equations can be used to remove t and get the trajectory of the particle:

As this is a quadratic equation in x, the particle follows a parabolic trajectory.