Difference between revisions of "Schrodinger equation"
| Line 15: | Line 15: | ||
-\frac{\hbar^2}{2m}\frac{\partial^2\psi}{\partial x^2}+V(x)\psi=i\hbar\frac{\partial \psi}{\partial t} | -\frac{\hbar^2}{2m}\frac{\partial^2\psi}{\partial x^2}+V(x)\psi=i\hbar\frac{\partial \psi}{\partial t} | ||
</math> | </math> | ||
| + | |||
| + | ===Derivation=== | ||
| + | The following derivation, likely one that Schrodinger followed himself, is a completely non-rigorous method which takes a more intuitive approach. Whatever ambiguities arose in this derivation because of its questionable assumptions were wiped out by subsequent experiments verifying again and again the equation's ability to predict probabilities of particle location<ref>French, A.P. and Taylor, E.F.. ''An Introduction to Quantum Physics''. CRC Press, Boca Raton, FL. Copyright MIT 1978.</ref>. | ||
| + | |||
| + | To start out, we assume that Planck and Einstein's quantized energy equation, <math>E = h\nu</math> where E is the energy, h is planck's constant, and nu is the frequency, is correct. We also assume that DeBroglie's wavelength of particles equation, <math>\lambda_dB = \frac{h}{p}</math> where lambda is the wavelength, h is planck's constant, and p is the momentum of the particle, is correct (these were indeed questionable assumptions during Schrodinger's time). Now we can rewrite the energy equation by multiplying and dividing the right hand side by <math>2 \pi</math> and turn it into | ||
| + | |||
| + | |||
| + | <math>E = \frac{h\omega}{2\pi}=\hbar\omega</math> | ||
| + | |||
| + | |||
| + | where omega is now the angular frequency. We can also rewrite DeBroglie's equation in the same way when divided by <math>2\pi</math>, turning it into | ||
| + | |||
| + | |||
| + | <math>\frac{\lambda}{2\pi} = \frac{\hbar}{p} or \frac{1}{k} = \frac{\hbar}{p}</math> | ||
| + | |||
| + | |||
| + | where k is the [[wavenumber]] of wavelength <math>\lambda_dB</math>. | ||
| + | |||
| + | Now, we can derive energy equations using classical Newtonian mechanics and plug our results in from the new developments above. Energy in classical terms is | ||
| + | |||
| + | |||
| + | <math>E = K_E + V_E = \frac{p^2}{2m} + V</math> | ||
| + | |||
| + | |||
| + | where | ||
| + | E = energy, <math>K_E = frac{1}{2}mv^2</math> (kinetic energy), <mathV_E = V</math> (potential energy) | ||
| + | |||
| + | Note that we've rewritten kinetic energy in terms of momentum, <math>p = mv</math>. Subbing in energy from the quantized energy equation and momentum from DeBroglie's equation, we obtain | ||
| + | |||
| + | |||
| + | <math>\hbar\omega = \frac{\hbar^2k^2}{2m} + V</math> | ||
| + | |||
| + | Notice this looks kind of like | ||
===Eigenvalue problems=== | ===Eigenvalue problems=== | ||
| Line 35: | Line 68: | ||
<math>E=\frac{\hbar^2 k^2}{2m}</math> | <math>E=\frac{\hbar^2 k^2}{2m}</math> | ||
| − | Physically, this corresponds to a wave | + | Physically, this corresponds to a wave traveling with a [[momentum]] given by <math>\hbar k</math>, where k can in principle take any value. |
===Particle in a box=== | ===Particle in a box=== | ||
| Line 45: | Line 78: | ||
<math>E_n=\frac{\hbar^2 k^2}{2m}=\frac{\hbar^2n^2\pi^2}{2m}</math> | <math>E_n=\frac{\hbar^2 k^2}{2m}=\frac{\hbar^2n^2\pi^2}{2m}</math> | ||
| + | |||
| + | |||
| + | ==References== | ||
| + | <small><references/></small> | ||
[[Category:Physics]] | [[Category:Physics]] | ||
Revision as of 22:41, September 28, 2009
The Schrodinger equation is a linear differential equation used in various fields of physics to describe the time evolution of quantum states. It is a fundamental aspect of quantum mechanics. The equation is named for its discoverer, Erwin Schrodinger.
Contents
Mathematical forms
General time-dependent form
The Schrodinger equation may generally be written
where 
is the imaginary unit,
is Planck's constant divided by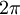 
, 
is the quantum mechanical state or wavefunction (expressed here in Dirac notation), and 
is the Hamiltonian operator.
The left side of the equation describes how the wavefunction changes with time; the right side is related to its energy. For the simplest case of a particle of mass m moving in a one-dimensional potential V(x), the Schrodinger equation can be written
Derivation
The following derivation, likely one that Schrodinger followed himself, is a completely non-rigorous method which takes a more intuitive approach. Whatever ambiguities arose in this derivation because of its questionable assumptions were wiped out by subsequent experiments verifying again and again the equation's ability to predict probabilities of particle location[1].
To start out, we assume that Planck and Einstein's quantized energy equation, 
where E is the energy, h is planck's constant, and nu is the frequency, is correct. We also assume that DeBroglie's wavelength of particles equation, 
where lambda is the wavelength, h is planck's constant, and p is the momentum of the particle, is correct (these were indeed questionable assumptions during Schrodinger's time). Now we can rewrite the energy equation by multiplying and dividing the right hand side by 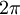and turn it into
where omega is now the angular frequency. We can also rewrite DeBroglie's equation in the same way when divided by 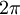, turning it into
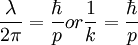
where k is the wavenumber of wavelength 
.
Now, we can derive energy equations using classical Newtonian mechanics and plug our results in from the new developments above. Energy in classical terms is
where
E = energy, 
(kinetic energy), <mathV_E = V</math> (potential energy)
Note that we've rewritten kinetic energy in terms of momentum, 
. Subbing in energy from the quantized energy equation and momentum from DeBroglie's equation, we obtain
Notice this looks kind of like
Eigenvalue problems
In many instances, steady-state solutions to the equation are of great interest. Physically, these solutions correspond to situations in which the wavefunction has a well-defined energy. The energy is then said to be an eigenvalue for the equation, and the wavefunction corresponding to that energy is called an eigenfunction or eigenstate. In such cases, the Schrodinger equation is time-independent and is often written
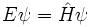
Here, E is energy, H is once again the Hamiltonian operator, and 
is the energy eigenstate for E.
One example of this type of eigenvalue problem is an electrons bound inside an atom.
Examples for the time-independent equation
Free particle in one dimension
In this case,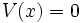 
and so we see that the solution to the Schrodinger equation must be
with energy given by
Physically, this corresponds to a wave traveling with a momentum given by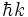 
, where k can in principle take any value.
Particle in a box
Consider a one-dimensional box of width a, where the potential energy is 0 inside the box and infinite outside of it. This means that must be zero outside the box. One can verify (by substituting into the Schrodinger equation) that
is a solution if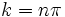 
where n is any integer. Thus, rather than the continuum of solutions for the free particle, for the particle in a box there is a set of discrete solutions with energies given by
References
- ↑ French, A.P. and Taylor, E.F.. An Introduction to Quantum Physics. CRC Press, Boca Raton, FL. Copyright MIT 1978.
