Talk:Axiom of Choice

Any proof which uses the axiom of choice can be transformed into a proof that doesn't. Granted, it will be a somewhat more complicated proof, but it always works, and that's a fact. That is the reason that AC is much less controversial these days than it was, in the early 1900s.

There is a complete explanation of the process and the proof that it's reliable here.

Also, the profoundly intuitive trichotomy is equivalent to AC, so be careful what you call controversial. BenjB 20:29, 27 January 2008 (EST)

Actually, the Axiom of Choice has been proven independent of ZF, so there is no such transformation of a proof. Otherwise, "prove" AC as follows:

1. Axiom of Choice | Reason: Axiom of Choice

Then transform it to not need AC. Result: AC proven in ZF,so ZFC=ZF. But AC proved independent of ZF. Therefore, no such transformation exists. QED SamSamson 21:50, 8 June 2008 (EDT)

This axiom actually can prove things which are (provably) unprovable without it; an example is Zorn's lemma. (Funnily enough, although Zorn is a guy's name, it's also a German word meaning "rage" ;-) --AMackenzie 14:29, 2 August 2008 (EDT)

The Axiom of Choice can also be used to "prove" absurdities, as explained by the entry here.--Aschlafly 14:52, 2 August 2008 (EDT)

It is a wonderful thing in mathematics when an absurdity is proven - it expands the consciousness. Either the "absurdity" isn't as absurd as once thought (the Earth is a ball, not a plain; there are just as many whole numbers as there are fractions), or some axiom needs to be rethought, or you've made a mistake. Whatever, you can learn from it.--AMackenzie 17:45, 2 August 2008 (EDT)

Banach-Tarski isn't absurd; it's just counterintuitive. -CSGuy 22:21, 2 August 2008 (EDT)

It is absurd. Indeed, the point of the "proof" was to show how absurd it is.--Aschlafly 23:14, 2 August 2008 (EDT)

It's absurd - no, it's not - yes, it is... As "being absurd" in this context is just a personal opinion, the phrase counter-intuitive seems to fit better. DiEb 10:28, 3 August 2008 (EDT)

I "prettied up" the quote from General Topology, but in context, Willard might not be the best person to quote after saying "many mathematicians reject the Axiom of Choice". Immediately before describing the axiom, he writes:

The following axiom is assumed by most mathematicians when they need it, to the unremitting disgust of a few.

And after the text quoted in the article:

The status of the axiom of choice bears some resemblance to that of the continuum hypothesis, with some differences. It, too, is known to be independant of the other axioms of set theory (that is, it or its negation can be consistently assumed), but it enjoys the status of an accepted part of the theory of sets in the minds of most modern mathematicians; that is, the intuition of almost all mathematicians now is that the axiom of choice should be assumed when needed without hestitation. Moreover, it is usually clearer that, where it is used, it is needed, so that its presence does not usually provoke the same frenzy of attempt to eliminate it.

Wandering 23:57, 2 August 2008 (EDT)

• I was unable to find references saying that the existence of a basis for every vector space and the existence of subsets of the real line without well-defined Lebesgue measure are equivalent to the Axiom of Choice. I did find references saying that the Axiom of Choice implies these, but that's not the same thing. -CSGuy 21:35, 26 November 2008 (EST)

The Axiom of Choice is not equivalent to the existence of non-Lebesgue measurable subsets of the real line and so I have removed this claim from the article. Intuitively one would not expect these to be equivalent. The existence of a non-Lebesgue measurable subset of the real line only says something about cardinals up to and including the continuum. However the Axiom of Choice makes a statement about all cardinals (in fact it is typically invoked when describing cardinals). AndyJM 14:27, 27 November 2008 (EST)

I got curious and actually looked into this. The Axiom of Choice does imply the existence of non-Lebesgue measurable subsets of the real line. However, the existence of non-Lebesgue measurable subsets of the real line doesn't imply the Axiom of Choice. I found a paper called "The Hahn-Banach theorem implies the existence of a non-Lebesgue measurable set" and will quote the abstract for those who don't want to download a 500+kb document:

So the other direction of the claim is apparently wrong, and AndyJM was right to remove it. He was blocked for "removing information from articles" by the same user who also re-inserted the apparently wrong claim. This is exactly why I oppose this "Block first" approach: How can we improve this encyclopedia when we can get blocked and banned by whoever thinks we're wrong? I suggest an unblock. --AlanS 18:38, 27 November 2008 (EST)

Alan, the Axiom of Choice yields many strange behaviors in mathematics. That's why it is still questioned and debated. If the statement is not equivalent to AC (which I will have to look deeper into to believe), then the correct behavior is not to remove the statement altogether but to change it to an implication. Edits that whitewash the Axiom of Choice's questionable role in mathematics are the sort of behavior you see at Wikipedia, not here (see Conservapedia:Critical Thinking in Math for a guide to our positions). I am reinstating the fact, but will tentatively change it to just a consequence of AC instead of an equivalence. -Foxtrot 18:50, 27 November 2008 (EST)

I have no problems with stating that it's a consequence of AC, but that doesn't change (1) that you first re-inserted the apparently wrong information, (2) that you claimed on your talk page that "[i]t is well known that you need AC to create a non-Lebesgue measurable set of real numbers" and (3) that you blocked two users over this content dispute, one of them for five years. --AlanS 18:57, 27 November 2008 (EST)

I reinserted valuable information that was lost by an editor intent to misrepresent the Axiom. If you persist in misrepresnting my actions in this matter, you may find yourself as the third Musketeer. -Foxtrot 19:01, 27 November 2008 (EST)

I have taken this to the Abuse Helpdesk. --AlanS 19:24, 27 November 2008 (EST)

I'll look into this further but upon first glance agree with Foxtrot. Wikipedia, not here, is the place to pretend falsely that the Axiom of Choice cannot be questioned. It is questioned, it is disfavored, and it does sometimes yield absurd results. Further comments welcome here, and if anyone thinks someone was improperly blocked then let's look at all his edits here.--Aschlafly 19:13, 27 November 2008 (EST)

Nobody claimed anything like that in this case. However, AndyJM removed a claim that was apparently wrong, and he was blocked for it. And BRichtigen was blocked for not simply ignoring this issue once Foxtrot lost interest. --AlanS 19:24, 27 November 2008 (EST)

AndyJM repeatedly deleted insights from entries, without explaining his deletions. That can be highly destructive if allowed to continue. We can debate the validity of his deletions, but at first glance AndyJM appears to me to have been wrong. Certainly his style was inappropriate in failing to explain or discuss his deletions. His block can be undone if you disagree, but more discussion would be needed about his specific edits.--Aschlafly 20:09, 27 November 2008 (EST)

Thank you, Andy, for considering my reasoning. The reason for the block of AndyJM wasn't simply his edits to this entry, but his removal of multiple portions of several mathematics entries. I had looked at a few and saw that he was removing good material without explanation and so he was being destructive. -Foxtrot 20:24, 27 November 2008 (EST)

Could you at least unblock BRichtigen? He already kicked off an analysis, but then you blocked him. I will paste the relevant exchange from your talk page:

Yes, of course, he was removing wrong information from articles

1. AC is implies the existence of non-Lebesgue-mb sets on an intervall, but isn't equivalent to it.
2. This, he outlined on the corresponding talk page
3. Next removal: An irrational number is formally defined to be the limit of a Cauchy sequence of rational numbers. A real number can be formally defined to be the limit of a Cauchy sequence of rational numbers. For example, a constant sequence is Cauchy and will lead to a rational number...
4. involvoling -> involving - no offense there, I presume
5. The cardinality of such a set would be denoted by the Hebrew letter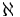: He was right to remove this, too: 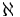without an index doesn't make much sense...
6. The continuum is called so because it was the first (and most prominent) continuous set studied by mathematicians. What does this mean? Remove it, I'd say...

All his entries were thoughtful and improve CP. --BRichtigen 16:41, 27 November 2008 (EST)

I disagree with his removals and your recent edits to the Obama article as well as your past history do not instill confidence in what you are saying. Typos can be fixed, but it's wrong to remove information along with the typos. -Foxtrot 16:44, 27 November 2008 (EST)

It's too late for me to do my own analysis right now, but I will see if I can add something tomorrow. In the meantime, you could do your part to show how AndyJM's edits were bad enough to warrant a five-year ban. We showed that at least one of his removals was entirely justified because it removed wrong information. I wouldn't be surprised if his other edits were okay, too. --AlanS 22:04, 27 November 2008 (EST)

And one for Andy...

• AndyJM repeatedly deleted insights from entries, without explaining his deletions.

It has been pointed out that (for example) his edit to this article was correct - the claim had been wrong. So it hadn't been an insight, but an error.

• That can be highly destructive if allowed to continue.

In my eyes, it's way more destructive to insert wrong information and to use block powers to intimidate and silence the opposition.

• We can debate the validity of his deletions, but at first glance AndyJM appears to me to have been wrong.

At least in this case, he had been right. And BRichtigen tried to discuss the other edits, but Foxtrot wasn't in the mood and instead won the discussion by means of blocking.

• Certainly his style was inappropriate in failing to explain or discuss his deletions.

He explained at least this deletion here, but Foxtrot removed the explanation for some reason instead of, you know, discussing about its validity. He also summed up his reasoning in the edit summaries at least.

• His block can be undone if you disagree, but more discussion would be needed about his specific edits.

It would be immensely helpful if you could unblock BRichtigen. He already started such a discussion (I pasted a part of it here), and his knowledge might prove to be useful. I can (and will) take a closer look myself tomorrow, but having another experienced editor handy would lighten my load. --AlanS 22:13, 27 November 2008 (EST)

What's the point of this edit: to illustrate that another axiom also results in an absurdity? It strikes me as wrong to try to justify the AOC by pointing out that an absurdity can be reached without using it also:

"Another seemingly absurd consequence of the Axiom of Choice is that there are subsets of the real line which do not have a well-defined Lebesgue measure. However, it is also possible to construct such a subset without using the Axiom of Choice.^[1]"

--Aschlafly 19:17, 27 November 2008 (EST)

All other examples in that section seem to REQUIRE the AC. The subset thing doesn't. My edit doesn't justify the AC, but it points out that the nature of that absurdity isn't a direct consequence of the AC - the AC can just be used to construct it easily. --AlanS 19:28, 27 November 2008 (EST)

But your distinction seems misplaced. The meaningful point is that AC proves an absurdity. It is irrelevant that another axiom may also produce the absurdity.--Aschlafly 19:46, 27 November 2008 (EST)

Even if the AC was completely blown out of the water somehow, the subset issue would still exist. In a section where practically every other example depends on the AC, that is a distinction worth pointing out. But I rephrased it again. Does this please you more? I'm not here to defend or fight, but the omission of the proven possibility of constructing the subsets without AC had been notable, so I fixed it. --AlanS 21:56, 27 November 2008 (EST)

1. ↑ "The Hahn-Banach theorem implies the existence of a non-Lebesgue measurable set"