Cross product
|
This article/section deals with mathematical concepts appropriate for late high school or early college. |
The cross product (or vector product) of two vectors in 3-space is itself a vector in 3-space and is written 
. The magnitude of the resulting vector is
where 
is the angle between the two vectors.
For two vectors 
and 
,
.
The direction of the cross product is normal to both of the vectors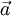 
and 
. Since there are two such directions the chosen one is defined by the right hand rule: with your right hand, point your fingers along the direction of the first vector and curl them towards the second vector. The direction your thumb points gives the direction of the cross product. This means that the cross product is anticommutative: 
.
The cross product is a convenient way to find the volume of a parallelepiped. One may simply take the cross product of two legs and then find the dot product of that vector with the remaining leg (assuming that the legs are vectors). So, a parallelepiped with sides 
and 
will have a volume,
, of:
Application
The cross product is used to find the torque and other parameters in physics, and is a clever way of finding the perpendicular vector to a plane.
Two of Maxwell's Equations make use of the cross product.
