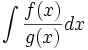Partial fractions in integration

This article/section deals with mathematical concepts appropriate for late high school or early college.

Integration by partial fractions is a technique in Calculus to facilitate the integration of a rational expression by partial fraction decomposition. Given an integral

where and are both polynomials, integration by partial fractions shows how to separate the problem into multiple integrals before integrating.

A 1st-Degree Denominator

These are a few methods of solving integrals with first degree denominators.

A 1st-Degree Denominator

Given:

Substitute

This means that if given an integral such as:

The steps can be skipped by using the general formula above get:

A Repeated 1st-Degree Denominator

The formula for integrals where a first degree polynomial denominator is raised to a power greater than one is much different than the formula above. Given:

Note that the above formula only works if .
This means that integrals such as

are now very easy:

A 2nd-Degree Denominator

2nd-Degree Denominators get more complicated, especially with those that do not factor.

A Reducible 2nd-Degree Polynomial Denominator

The first step is to factor the denominator as much as possible and get the form of the partial fraction decomposition. Doing this gives,

This allows the denominator to split the fraction in to sums by cross multiplying the denominators,

Therefore, the problem can be restated,

Now it is possible to solve for A and B by substituting x with a value that allows the term to go to 0. For example,

Let :,

Let :,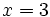

Then plug in the values of A and B and get,

Now solve the integral.

See also

• Calculus
• Integral
• Methods of integration